Deterministic Chaos

Cycles

How can we locate the points belonging to an n-cycle?

For example, consider the 2-cycle consisting of the points x = a and x = b.

From the graph it is easy to see T(a) = b and T(b) = a. Consequently,

T(T(a)) = T(b) = a	and	T(T(b)) = T(a) = b
That is,
T2(a) = a	and	T2(b) = b

In other words, if x = a and x = b belong to a 2-cycle for T(x), both are fixed points for T²(x) = T(T(x)).

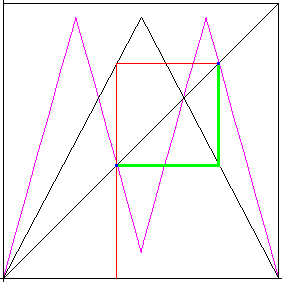
This is useful, because we know how to locate fixed points of any function graphically: look for the intersections of the graph of T²(x) (purple below) with the diagonal.

Click the picture to animate.

Note the fixed points of T(x) also are fixed points of T²(x).

This is hardly a surprise:

if T(c) = c,

then T²(c) = T(T(c)) = T(c) = c.

This observation has obvious generalizations. For example,

Do you see the general pattern?

Return to Cycles.