Deterministic Chaos
Logistic Map Cycles
We have seen that points of an
n-cycle of L(x) are fixed points of Ln(x). So we
look at graphs of some compositions of the logistic map for various
s-values.
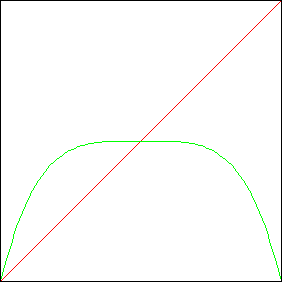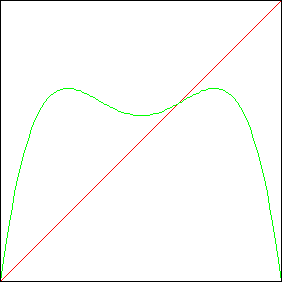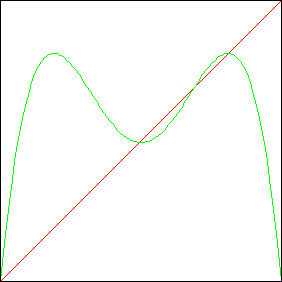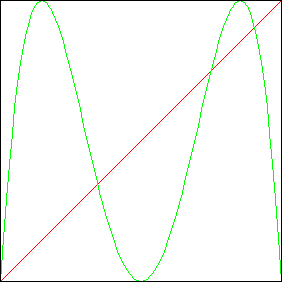
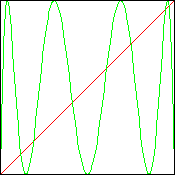
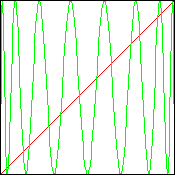
Here we see the s = 4 pictures for
L2(x), L3(x), and L4(x). Click on each
picture for an animation of s going from 2 to 4 in steps of 0.25. Click an animation to stop.
A careful comparison of these three animations reveals cycles
arise in two different ways.
The 2-cycle appears where one of the
fixed points of L(x) becomes unstable.
But the 3-cycle arises
from nothing.
Maybe the difference has to do with even and odd cycles.
No, looking at the 4-cycle animation shows two 4-cycles:
the first appears where the 2-cycle becomes unstable,
the second arises from nothing.
In fact, these are the two mechanisms for producing
new cycles. The first is called a
period-doubling bifurcation, the second a
tangent bifurcation.
Here is a brief description of the geneology
of cycles.
Return to cycles.