Deterministic Chaos

6.N. Period-Doubling Scaling and the Feigenbaum Constant

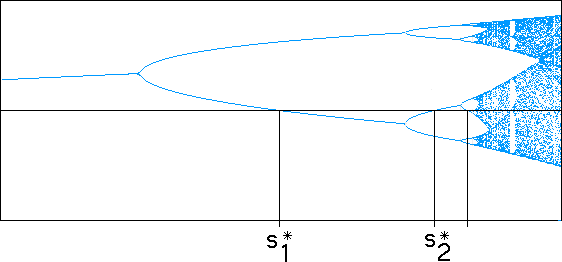
Here are the first few superstable points. For example,

at s*₀ the point x = 1/2 is a fixed point,

at s*₁ the point x = 1/2 belongs to a 2-cycle,

at s*₂ the point x = 1/2 belongs to a 4-cycle,

and so on.

Here are the first 14 numerical values.

s*0	= 2.000000000000000000
s*1	= 3.236067977499789696
s*2	= 3.498561699327701520
s*3	= 3.554643880189573995
s*4	= 3.566667594798299166
s*5	= 3.569243531637110338
s*6	= 3.569795293749944621
s*7	= 3.569913465422348515
s*8	= 3.569938774233305491
s*9	= 3.569944194608064931
s*10	= 3.569945355486468581
s*11	= 3.569945604111078447
s*12	= 3.569945657358856505
s*13	= 3.569945668762899979
s*14	= 3.569945671205296863

We observe the superstable points are getting closer and closer together. In fact, the sequence of s*_n converges to the Myerberg point, s*_infinity, the same point to which the period-doubling s_i converge.

Return to Period-Doubling Scaling and the Feigenbaum Constant.