Deterministic Chaos

6.N. Period-Doubling Scaling and the Feigenbaum Constant

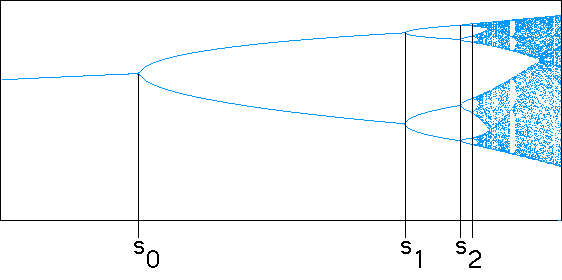
Suppose we note the s-values where successive period-doubling bifurcations occur.

Call s₀ the value where the nonzero fixed point becomes unstable and a stable 2-cycle appears,

s₁ the value where this 2-cycle becomes unstable and a stable 4-cycle appears,

s₂ the value where this 4-cycle becomes unstable and a stable 8-cycle appears,

and so on.

The first few points are illustrated in the picture.

Unfortunately, calculating the bifurcation values s_i to high accuracy is very difficult. Much easier is to determine the superstable values s*_i, the s-values in each periodic window where x = 1/2 belongs to the cycle. This is a reasonable approach because the sequences of period-doubling and superstable values share many properties.

Here are the first 14 supersable s*i

The simplest approach to detect a scaling relation between the s*_n is to calculate how the dstance between them changes. That is, find the ratio of successive differences.

Here are the ratios of the differnces of the first few supersable s*i.

It's interesting that the ratios of successive diatances converge, but is this enough to earn Feigenbaum a MacArthur grant? The real interest in this constant comes from its universality.

There is another constant associated with this scaling.

Return to Deterministic Chaos.